“The Comparative Statics of Sorting“, with Axel Anderson (Accepted at American Economic Review)

We create a general and tractable theory of increasing sorting in pairwise matching models with monetary transfers. Our partial order, positive quadrant dependence, subsumes Becker (1973) as the extreme cases with most and least sorting. It implies sorting by correlation of matched partners, or distance between partners.

Our theory turns on synergy — the cross partial difference or derivative of match production. This reflects basic economic forces: diminishing returns, technological convexity, insurance, and match learning dynamics. We prove that sorting increases if match synergy globally increases, and is also cross-sectionally monotone or single-crossing. Our theorems shed light on major economics sorting papers, affording immediate proofs and new insights. They open the door to fast predictions for new pairwise sorting models in economics.

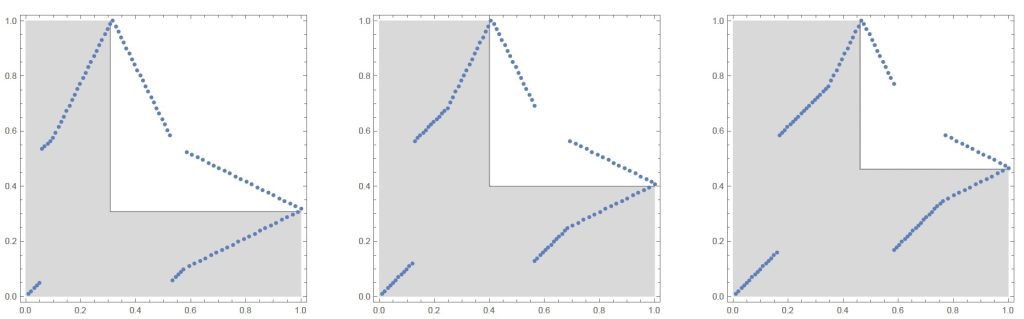
We prove that sorting increases if match synergy globally increases, and is also cross-sectionally monotone or single-crossing. Our theorems shed light on major economics sorting papers, affording immediate proofs and new insights. They open the door to fast predictions for new pairwise sorting models in economics.

• For optimal matching plots in the paper, pictures are generated by Mathematica. The README is here.
• For figure EHK_2Period_Fig12, the compressed Mathematica file is here.
• For figure MinSynergy_Fig4, the compressed Mathematica file is here.
• For figure DiminishingRet_Fig9, the compressed Mathematica file is here.
• For figure SmoothKM_Fig10, the compressed Mathematica file is here.
• For figure SerfesPA_Fig11, the compressed Mathematica file is here.
• For figure TypeShifts_Fig8, the compressed Mathematica file is here.