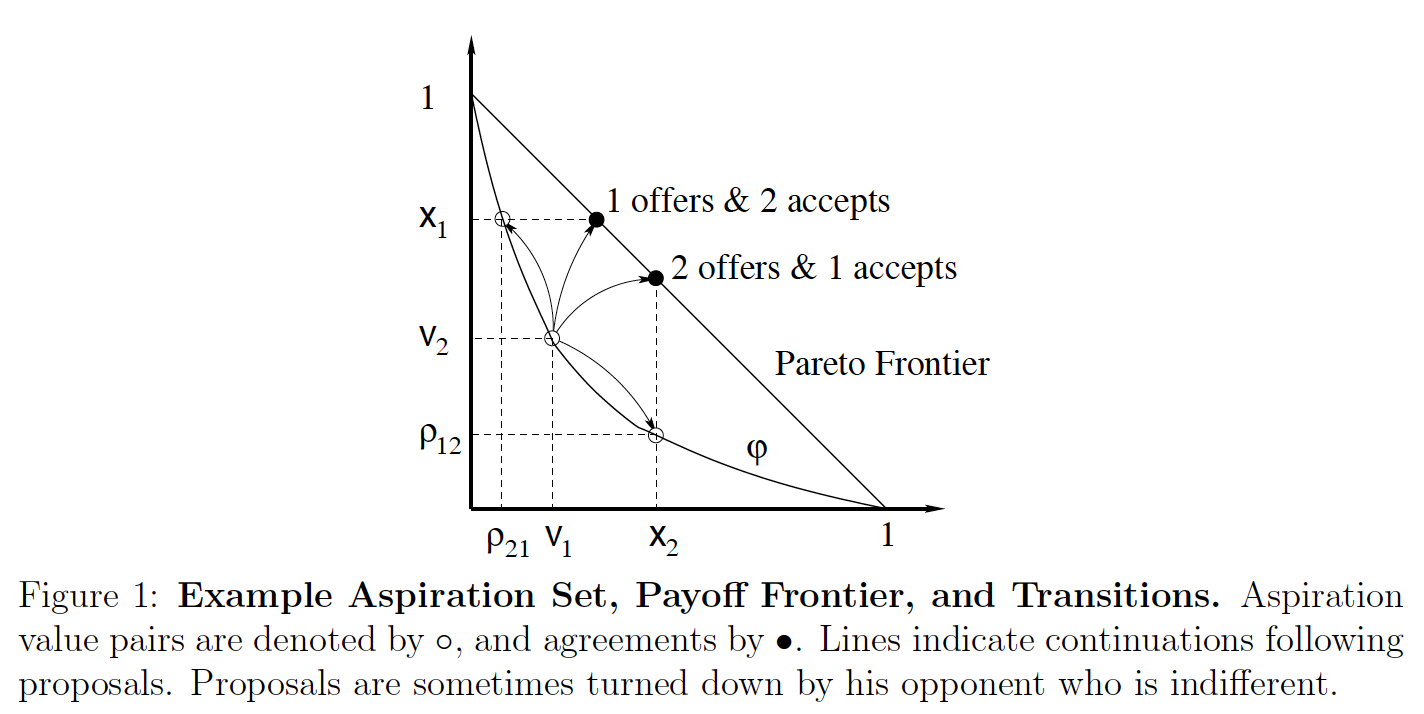
Aspirational Bargaining, Smith and Stacchetti (Michigan working paper, 2003)
Assume that two impatient individuals wish to divide a unit pie. This paper offers a noncooperative resolution of this classic complete information bargaining problem without procedures. To do away with the reliance on exogenous procedures, we formulate the game in continuous time, with unrestricted timing and content of offers. To deal with the multiple equilibria, we employ a standard refinement in repeated games that admits a simple behavioural interpretation: Reprising experimental work from 1960, we introduce and explore aspirational equilibrium — a Markovian refinement of subgame perfection where behaviour is governed by aspiration values (expected payoffs). The analysis is tractable, and generates many intuitive aspects of bargaining. We find that discounted aspiration values form a martingale, and thereby compute bounds on the expected bargaining duration. We also deduce some simple implications about consecutive offers, and relate delay times, offers, and acceptance rates. Finally, we draw into question a traditional comparative static: Ceteris paribus, more impatient players can expect more of the pie.
See my earlier “I Have a Dream” manuscript: “Rethinking Bargaining Theory” (1998 MIT working paper)